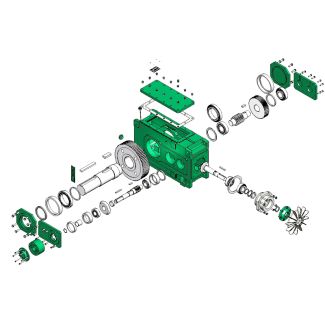
Bevel-helical speed reducer B4 aft seal Z QD Side Dual radial shaft sealSide Sp B4-HV-14-A
In stock
SKU
B4-HV-14-A
$58,607.14
Flender/Flender Gear Units/Bevel-helical speed reducer B4
tor. However, the thermal efciency of an array of these small collectors is lower than that of single unit (. Thus, it leads to an erroneous design. The thermalperformance of the total collector system also depends on the conguration of
Thus, it leads to an erroneous design. The thermalperformance of the total collector system also depends on the conguration of  the smallcollector units in the array. Use of separate units to constitute the total system gives largethermal losses from the
the smallcollector units in the array. Use of separate units to constitute the total system gives largethermal losses from the  edges that could be minimized to large extent using an integratedarray of collector modules. In general, the total useful energy
edges that could be minimized to large extent using an integratedarray of collector modules. In general, the total useful energy  gain of collector is expressed as follows (: /H1 wC (to/H1ta)/H1AcFR[()eIT/H1UL(ti/H1ta)] ( where Quis the total useful energy available from the collector (/m,wis the mass ow rate of air (kg/), Cpis the specic heat of air (kJ/kg /H,toandtaare the collector outlet and ambient temperatures ( ),ACis the collector area (m,FRis the collector heat removal factor, ( )eis the effective transmittanceabsorptance product, ITis the insolation on the tilted collector (/m,ULis the overall heat transfer coefcient (/ m2K/H, and tiis the temperature of air at the collector inlet ( ). Figure 1 shows schematic of an integrated array of collectors (2 m that com- prises ten collectors having an area of 2 m2for each. The thermal efciency ( ) ft system can be dened as follows: /H1wC (to/H1ti) AcIT/H1( The efciency of the system can be improved using suitable airow (Fig. . The prediction of outlet air temperature under various organizations of airow can be madeby an iterative procedure (. The success or accuracy of the predictive model, however, Fig. 1 Schematic of different airow congurations with the integrated array of collectors. 1 Das and Chakraverty Fig. 1 Performance of an integrated array of collectors for different airow congurations. (From Ref. 2.) depends on the realistic estimation of the heat-transfer coefcients, which, in turn, are functions of the absorber geometry, airow geometry, and tilt angle of the collector. The following multiple regression equation h
gain of collector is expressed as follows (: /H1 wC (to/H1ta)/H1AcFR[()eIT/H1UL(ti/H1ta)] ( where Quis the total useful energy available from the collector (/m,wis the mass ow rate of air (kg/), Cpis the specic heat of air (kJ/kg /H,toandtaare the collector outlet and ambient temperatures ( ),ACis the collector area (m,FRis the collector heat removal factor, ( )eis the effective transmittanceabsorptance product, ITis the insolation on the tilted collector (/m,ULis the overall heat transfer coefcient (/ m2K/H, and tiis the temperature of air at the collector inlet ( ). Figure 1 shows schematic of an integrated array of collectors (2 m that com- prises ten collectors having an area of 2 m2for each. The thermal efciency ( ) ft system can be dened as follows: /H1wC (to/H1ti) AcIT/H1( The efciency of the system can be improved using suitable airow (Fig. . The prediction of outlet air temperature under various organizations of airow can be madeby an iterative procedure (. The success or accuracy of the predictive model, however, Fig. 1 Schematic of different airow congurations with the integrated array of collectors. 1 Das and Chakraverty Fig. 1 Performance of an integrated array of collectors for different airow congurations. (From Ref. 2.) depends on the realistic estimation of the heat-transfer coefcients, which, in turn, are functions of the absorber geometry, airow geometry, and tilt angle of the collector. The following multiple regression equation h| Model Type | Bevel-helical speed reducer B4 |
|---|---|
| Gear Type | Bevel Helical Gear |
| Weight (kg) | 2735.000000 |
| Ratio Range | 1 : 100…400 |
| Low Speed Output | Hollow shaft with keyway acc. to DIN 6885/1 |
| Nominal Torque | 113000 Nm |
| Mounting Arrangements | Vertical mounting position |
| Manufacturer | Siemens AG |
| Country of Manufacture | Palau |
| Data Sheet & Drawings | Bevel-helical speed reducer B4 aft seal Z QD Side Dual radial shaft sealSide Sp B4-HV-14-A |