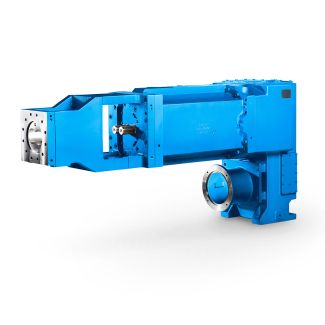
er filters are provided as the standard because t B4-DV15A Bevel-helical speed reducer B4
In stock
SKU
B4-DV15A
$77,785.71
Flender/Flender Gear Units/Bevel-helical speed reducer B4
uid. At higher tempera- tures, the pore pressure may rise substantially and cause hydrodynamically driven owof vapor that, in turn, may cause pressure-driven ow of liquid in the porous material. Fig. 8 Characteristic drying rate curve. Grain Drying Basics 1
may cause pressure-driven ow of liquid in the porous material. Fig. 8 Characteristic drying rate curve. Grain Drying Basics 1  For solids with continuous pores, surfacetension-driven ow (capillary ow) may occur as result of capillary forces caused by the interfacial
For solids with continuous pores, surfacetension-driven ow (capillary ow) may occur as result of capillary forces caused by the interfacial  tension between the waterand the solid. In the simplest model, modied form of the Poiseuille ow can be usedin conjunction
tension between the waterand the solid. In the simplest model, modied form of the Poiseuille ow can be usedin conjunction  with the capillary force equation to estimate the rate of drying. Geankopolis( has shown that such model predicts that the drying rate in the falling rate period is proportional to the free-moisture content in the solid. At low, solid moisture contents, however, the diffusion model may be more appropriate. The moisture ux caused by capillarity can be expressed in terms of the product of liquid conductivity parameter and moisture gradient. Here, the governing equation hasthe same form as the diffusion equation. For certain materials and under conditions such as those encountered in freeze- drying, receding-front model involving moving boundary between dry and wet zones often describes the mechanism of drying much more realistically than doesthe simple liquid diffusion or capillarity model. Examination of the freeze-drying of thin slab indicates that the rate of drying is dependent on the rate of heat transfer to the drywet interface and the mass transfer resistance offered by the porous dry layer topermeation of the vapor that sublimes from the interface. Because of the low pressure encountered in freeze-drying, Knudsen diffusion may be signicant. Liapis and Marchello ( have discussed models of freeze-drying involving both unbound and bound mois- ture. When drying materials are under intense-drying conditions, diffusion or capillarity models generally do not apply. If evaporation can occur within the material, there is adanger of the so-called
with the capillary force equation to estimate the rate of drying. Geankopolis( has shown that such model predicts that the drying rate in the falling rate period is proportional to the free-moisture content in the solid. At low, solid moisture contents, however, the diffusion model may be more appropriate. The moisture ux caused by capillarity can be expressed in terms of the product of liquid conductivity parameter and moisture gradient. Here, the governing equation hasthe same form as the diffusion equation. For certain materials and under conditions such as those encountered in freeze- drying, receding-front model involving moving boundary between dry and wet zones often describes the mechanism of drying much more realistically than doesthe simple liquid diffusion or capillarity model. Examination of the freeze-drying of thin slab indicates that the rate of drying is dependent on the rate of heat transfer to the drywet interface and the mass transfer resistance offered by the porous dry layer topermeation of the vapor that sublimes from the interface. Because of the low pressure encountered in freeze-drying, Knudsen diffusion may be signicant. Liapis and Marchello ( have discussed models of freeze-drying involving both unbound and bound mois- ture. When drying materials are under intense-drying conditions, diffusion or capillarity models generally do not apply. If evaporation can occur within the material, there is adanger of the so-called| Model Type | Bevel-helical speed reducer B4 |
|---|---|
| Gear Type | Bevel Helical Gear |
| Weight (kg) | 3630.000000 |
| Ratio Range | 1 : 80…315 |
| Low Speed Output | Hollow shaft with shrink disk |
| Nominal Torque | 153000 Nm |
| Mounting Arrangements | Vertical mounting position |
| Manufacturer | Flender Siemens |
| Country of Manufacture | Syria |
| Data Sheet & Drawings | er filters are provided as the standard because t B4-DV15A Bevel-helical speed reducer B4 |