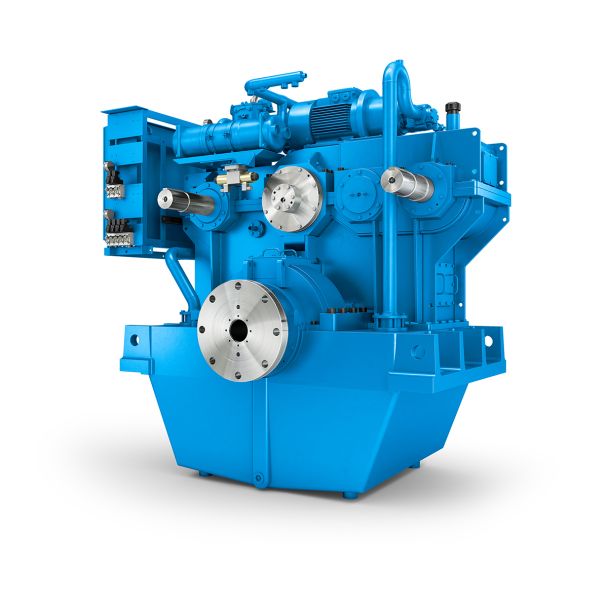
H4-CV-28B sed The standard material for housings is lamella Helical gear units H4
In stock
SKU
H4-CV-28B
$282,857.14
Flender/Flender Gear Units/Helical gear units H4
he wet-bulb temperature is independentof the geometry of the drying owing to the analogy between heat and mass transfer. The drying rate in the falling rate period() is function of (orX ) and must be determined experimentally for given material
drying rate in the falling rate period() is function of (orX ) and must be determined experimentally for given material  being dried in given type of dryer. If the drying rate curve ( Nvs.) is known, the total drying time
being dried in given type of dryer. If the drying rate curve ( Nvs.) is known, the total drying time  required to reduce the solid moisture content from X1toX2can be simply calculated by: td/H1/H1/H2X2 X1Ms AdX ( Table 7 lists
required to reduce the solid moisture content from X1toX2can be simply calculated by: td/H1/H1/H2X2 X1Ms AdX ( Table 7 lists  expressions for the drying time for constant rate, linear falling rates, and falling rate controlled by liquid diffusion of water in thin slab. The subscripts andfrefer to the constant and falling rate periods, respectively. The total drying time is, of course, sum of drying times in two succeeding periods. Different analytical expres-sions are obtained for the drying times fdepending on the functional form of Nor the model used to describe the falling rate (.., liquid diffusion, capillary, or evaporation- condensation). For some solids, receding front model (wherein the evaporating surfacerecedes into the drying solid) yielods good agreement with experimental observations.The principal goal of all falling rate drying models is to allow reliable extrapolation ofdrying kinetic data over various operating conditions and product geometries. The expression for fin Table 7 using the liquid diffusion model (Ficks second law of diffusion form applied to diffusion in solids with no real fundamental basis) is obtained by analytically solving the following partial differential equation: Xf /H1DL2Xf x2( subject to the following initial and boundary conditions: Xf/H1Xi, everywhere in the slab at /H1 Xf/H1, at /H1a(top, evaporating surface), and ( Xf /H1, at /H1 (bottom, nonevaporating surface) Grain Drying Basics 1 Table 7 Drying Times for Various Drying Rate Models Model Drying time td/H1Drying time to reach nal moisture content X2Kinetic model, /H1/H1Ms AdX dt from initial moisture content X1 /H1N
expressions for the drying time for constant rate, linear falling rates, and falling rate controlled by liquid diffusion of water in thin slab. The subscripts andfrefer to the constant and falling rate periods, respectively. The total drying time is, of course, sum of drying times in two succeeding periods. Different analytical expres-sions are obtained for the drying times fdepending on the functional form of Nor the model used to describe the falling rate (.., liquid diffusion, capillary, or evaporation- condensation). For some solids, receding front model (wherein the evaporating surfacerecedes into the drying solid) yielods good agreement with experimental observations.The principal goal of all falling rate drying models is to allow reliable extrapolation ofdrying kinetic data over various operating conditions and product geometries. The expression for fin Table 7 using the liquid diffusion model (Ficks second law of diffusion form applied to diffusion in solids with no real fundamental basis) is obtained by analytically solving the following partial differential equation: Xf /H1DL2Xf x2( subject to the following initial and boundary conditions: Xf/H1Xi, everywhere in the slab at /H1 Xf/H1, at /H1a(top, evaporating surface), and ( Xf /H1, at /H1 (bottom, nonevaporating surface) Grain Drying Basics 1 Table 7 Drying Times for Various Drying Rate Models Model Drying time td/H1Drying time to reach nal moisture content X2Kinetic model, /H1/H1Ms AdX dt from initial moisture content X1 /H1N| Model Type | Helical gear units H4 |
|---|---|
| Gear Type | Helical Gear |
| Weight (kg) | 13200.000000 |
| Ratio Range | 1 : 112…400 |
| Low Speed Output | Solid shaft without parallel key |
| Nominal Torque | 1400000 Nm |
| Mounting Arrangements | Vertical mounting position |
| Manufacturer | N.V. Flender Belge S.A. |
| Country of Manufacture | China |
| Data Sheet & Drawings | H4-CV-28B sed The standard material for housings is lamella Helical gear units H4 |