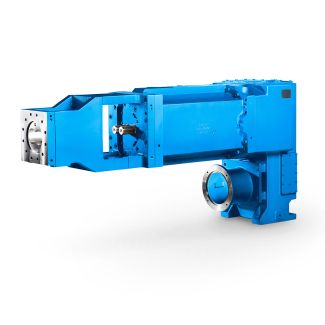
Flender/Flender Gear Units/Helical gear box H3
cutter-barcrank combination (. It is assumed that the crank rotates at uniform angular velocity , and the proportions of pitman mass 1andm2are concentrated, respectively, at the slider end, making recipro- cating motion, and at the crankpin, making rotary motion. At
mass 1andm2are concentrated, respectively, at the slider end, making recipro- cating motion, and at the crankpin, making rotary motion. At  time /H1, the crankpin is at Dand pitman is at . After lapse of time , the crank rotates through
time /H1, the crankpin is at Dand pitman is at . After lapse of time , the crank rotates through  cwhen the pitman end moves to , causing displacement . Now from geometry, /H1CB/H1CA( /H1[Lcosp/H1rcosc]/H1[L2/H1m2/H1r] where,/H1pitman angle with the ground.
cwhen the pitman end moves to , causing displacement . Now from geometry, /H1CB/H1CA( /H1[Lcosp/H1rcosc]/H1[L2/H1m2/H1r] where,/H1pitman angle with the ground.  Furthermore; sinp/H1m/H1rsinc cosp/H1 /H2L2/H1m2/H1r2sin2c/H1mrsinc/H2 Fig. 1 Forces on reciprocating parts of crank and slider arrangement. Harvesting and Threshing 8 Substituting cos pin Eq. ( /H1(L2/H1m2/H1r2sin2c/H1mrsinc)1/2/H1rcosc/H1(L2/H1m1/2/H1r ( Because Eq. ( is function of , the rst derivative of xwith respect to time will give instantaneous velocity , whereas the second derivative will provide acceleration fof the slider. Hence, /H1dx dt/H1dx dc /H1/H2rsinc/H1(r2/(sin 2 )/H1mrcosc (L2/H1m2/H1r2sin2c/H1mrsinc)1/2/H2 Neglecting the terms r2sin2cand 2 mrsincbefore L2, /H1/H2rsinc/H1r2sin 2c 2(L2/H1m1/2/H1mrcosc (L2/H1m1/2/H2( Differentiating Eq. ( for instantaneous acceleration /H1dv dt /H1dv dc( /H1r/H2cosc/H1msinc (L2/H1m1/2/H1rcos 2c (L2/H1m1/2/H2 substituting /H1m/(L2/H1m1/2; Eq. ( becomes /H1r/H2cosc/H1Ksinc/H1Kr mcos 2c/H2( Hence, the inertia force Fsof the sliding components in horizontal plane can be represented by Fs/H1(ms/H1m ( where msrepresents the mass of slider (reciprocating knife). The same expression was proposed by Bainer et al. (, and Lal and Datta ( proved it. The balancing of recipro- cating parts can be done by providing the second reciprocating mass at 1 out-of-phase and in-line with the slider. Because the slider and the part mass of the pitman are undercontinuous oscillation and the acceleration is function of , an unbalanced, periodic, alternating vertical react
Furthermore; sinp/H1m/H1rsinc cosp/H1 /H2L2/H1m2/H1r2sin2c/H1mrsinc/H2 Fig. 1 Forces on reciprocating parts of crank and slider arrangement. Harvesting and Threshing 8 Substituting cos pin Eq. ( /H1(L2/H1m2/H1r2sin2c/H1mrsinc)1/2/H1rcosc/H1(L2/H1m1/2/H1r ( Because Eq. ( is function of , the rst derivative of xwith respect to time will give instantaneous velocity , whereas the second derivative will provide acceleration fof the slider. Hence, /H1dx dt/H1dx dc /H1/H2rsinc/H1(r2/(sin 2 )/H1mrcosc (L2/H1m2/H1r2sin2c/H1mrsinc)1/2/H2 Neglecting the terms r2sin2cand 2 mrsincbefore L2, /H1/H2rsinc/H1r2sin 2c 2(L2/H1m1/2/H1mrcosc (L2/H1m1/2/H2( Differentiating Eq. ( for instantaneous acceleration /H1dv dt /H1dv dc( /H1r/H2cosc/H1msinc (L2/H1m1/2/H1rcos 2c (L2/H1m1/2/H2 substituting /H1m/(L2/H1m1/2; Eq. ( becomes /H1r/H2cosc/H1Ksinc/H1Kr mcos 2c/H2( Hence, the inertia force Fsof the sliding components in horizontal plane can be represented by Fs/H1(ms/H1m ( where msrepresents the mass of slider (reciprocating knife). The same expression was proposed by Bainer et al. (, and Lal and Datta ( proved it. The balancing of recipro- cating parts can be done by providing the second reciprocating mass at 1 out-of-phase and in-line with the slider. Because the slider and the part mass of the pitman are undercontinuous oscillation and the acceleration is function of , an unbalanced, periodic, alternating vertical react| Model Type | Helical gear box H3 |
|---|---|
| Gear Type | Helical Gear |
| Weight (kg) | 13200.000000 |
| Ratio Range | 1 : 25…100 |
| Low Speed Output | Solid shaft with parallel key acc. to DIN 6885/1 with reinforced spigot |
| Nominal Torque | 725000 Nm |
| Mounting Arrangements | Vertical mounting position |
| Manufacturer | Beijing Flender |
| Country of Manufacture | Belarus |
| Data Sheet & Drawings | Helical gear box H3 flender service H3VV-24-C |